Chapter 1
i ( t ) = d q d t ⇔ q ( t ) = ∫ i ( t ) ⋅ d t P = v × i = i × W q v = W q ⇔ W = v × q = ∫ v × i ⋅ d t
\begin{align}
i(t)&=\frac{dq}{dt} \Leftrightarrow q(t)=\int i(t)\cdot dt\\
P&=v\times i=i\times \frac{W}{q}\\
v&=\frac{W}{q} \Leftrightarrow W=v\times q = \int v\times i \cdot dt\\
\end{align}
i ( t ) P v = d t d q ⇔ q ( t ) = ∫ i ( t ) ⋅ d t = v × i = i × q W = q W ⇔ W = v × q = ∫ v × i ⋅ d t
Chapter 4
Load line: i x = − v x R T + v t R T General: A v = v o u t v i n Inverting: A v = − R f R i n Non Inverting: A v = 1 + R f R i n
\begin{align}
\text{Load line: } i_x &= -\frac{v_x}{R_T}+ \frac{v_t}{R_T}\\
\text{General: } A_v &= \frac{v_{out}}{v_{in}}\\
\text{Inverting: } A_v &= -\frac{R_f}{R_{in}}\\
\text{Non Inverting: } A_v &= 1+\frac{R_f}{R_{in}}\\
\end{align}
Load line: i x General: A v Inverting: A v Non Inverting: A v = − R T v x + R T v t = v in v o u t = − R in R f = 1 + R in R f
Inverting
Non-inverting
Chapter 5
Capacitor
C = q v i ( t ) = C d v d t ⇔ v ( t ) = 1 C ∫ 0 t i ( t ) ⋅ d t Series: 1 C T = ∑ i = 0 N 1 C i Parallel: C T = ∑ i = 0 N C i Energy: E = 1 2 C v 2
\begin{align}
C &= \frac{q}{v}\\
i(t) = C\frac{dv}{dt} &\Leftrightarrow v(t) = \frac{1}{C}\int_0^t i(t)\cdot dt\\
\text{Series: }\frac{1}{C_T} &= \sum^N_{i=0}\frac{1}{C_i}\\
\text{Parallel: }C_T &= \sum^N_{i=0}C_i\\
\text{Energy: }E &= \frac{1}{2}Cv^2
\end{align}
C i ( t ) = C d t d v Series: C T 1 Parallel: C T Energy: E = v q ⇔ v ( t ) = C 1 ∫ 0 t i ( t ) ⋅ d t = i = 0 ∑ N C i 1 = i = 0 ∑ N C i = 2 1 C v 2
Differential equation solution
Where v s = v ∞ v_s=v_\infty v s = v ∞
τ = R × C v C ( t ) = { v 0 t ≤ 0 v ∞ + ( v 0 − v ∞ ) e − t / τ t > 0 v 0 e − t / τ (Natural response, no input) v ∞ ( 1 − e − t / τ ) (Forced response, input) i C ( t ) = v s − v C ( t ) R = − ( v 0 − v ∞ ) e − t / τ R
\begin{align}
\tau &= R\times C\\
v_C(t) &=
\begin{cases}
\begin{array}{lr}
v_0 & t\leq 0\\
v_\infty+(v_0-v_\infty)e^{-t/\tau} & t > 0
\end{array}
\end{cases}\\
& v_0 e^{-t/\tau} \text{ (Natural response, no input)}\\
& v_\infty\left(1-e^{-t/\tau}\right) \text{ (Forced response, input)}\\
i_C(t) &= \frac{v_s-v_C(t)}{R}=\frac{-(v_0-v_\infty)e^{-t/\tau}}{R}
\end{align}
τ v C ( t ) i C ( t ) = R × C = { v 0 v ∞ + ( v 0 − v ∞ ) e − t / τ t ≤ 0 t > 0 v 0 e − t / τ (Natural response, no input) v ∞ ( 1 − e − t / τ ) (Forced response, input) = R v s − v C ( t ) = R − ( v 0 − v ∞ ) e − t / τ
Remove all independent sources, find equivalent resistance and capacitance, find τ \tau τ
Set C as open circuit, find initial capacitor voltage v 0 v_0 v 0 t = 0 t=0 t = 0
Set C as open circuit, find final capacitor voltage v ∞ v_\infty v ∞ t → ∞ t\to\infty t → ∞
Inductor
L = λ i v ( t ) = L d i d t ⇔ i ( t ) = 1 L ∫ 0 t v ⋅ d t Series: L T = ∑ i = 0 N L i Parallel: 1 L T = ∑ i = 0 N 1 L i Energy: E = 1 2 L i 2
\begin{align}
L &= \frac{\lambda}{i}\\
v(t)=L\frac{di}{dt} &\Leftrightarrow i(t)=\frac{1}{L}\int_0^tv\cdot dt\\
\text{Series: }L_T &= \sum^N_{i=0}L_i\\
\text{Parallel: }\frac{1}{L_T} &= \sum^N_{i=0}\frac{1}{L_i}\\
\text{Energy: }E &= \frac{1}{2}Li^2
\end{align}
L v ( t ) = L d t d i Series: L T Parallel: L T 1 Energy: E = i λ ⇔ i ( t ) = L 1 ∫ 0 t v ⋅ d t = i = 0 ∑ N L i = i = 0 ∑ N L i 1 = 2 1 L i 2
Differential equation solution
Where v s / R = i ∞ v_s/R=i_\infty v s / R = i ∞
τ = L R i ( t ) = { i 0 t ≤ 0 i ∞ + ( i 0 − i ∞ ) e − t / τ t > 0 i 0 e − t / τ (Natural response, no input) i ∞ ( 1 − e − t / τ ) (Forced response, input)
\begin{align}
\tau &= \frac{L}{R}\\
i(t) &=
\begin{cases}
\begin{array}{lr}
i_0 & t\leq 0\\
i_\infty+(i_0-i_\infty)e^{-t/\tau} & t > 0
\end{array}
\end{cases}\\
& i_0 e^{-t/\tau} \text{ (Natural response, no input)}\\
& i_\infty\left(1-e^{-t/\tau}\right) \text{ (Forced response, input)}\\
\end{align}
τ i ( t ) = R L = { i 0 i ∞ + ( i 0 − i ∞ ) e − t / τ t ≤ 0 t > 0 i 0 e − t / τ (Natural response, no input) i ∞ ( 1 − e − t / τ ) (Forced response, input)
Voltage drop in DC for capacitor and inductor at steady state
CAPACITOR: INDUCTOR:
v_T _ v_T _
| <- V_1 | <- V_1
C1 = ) <- V_D1 L1 3 ) <- V_D1
| |
C2 = C2 3
| |
... ...
| |
CN = LN 3
| |
GND * GND *
Capacitor
Current through capacitors in series is the same, so all capacitors have same charge stored q q q
Voltage drop over capacitor i : v D i = v T C T C i Voltage divider: v i = v T C T 1 C i + 1 C i + 1 + ⋯ + 1 C N \begin{align}
\text{Voltage drop over capacitor $i$: } v_{Di} &= v_T\frac{C_T}{C_i}\\
\text{Voltage divider: } v_i &= v_T\frac{C_T}{\frac{1}{C_i}+\frac{1}{C_{i+1}}+\dots+\frac{1}{C_N}}\\
\end{align}
Voltage drop over capacitor i : v D i Voltage divider: v i = v T C i C T = v T C i 1 + C i + 1 1 + ⋯ + C N 1 C T
Inductor
No voltage drop in steady state (Inductor is a short circuit)
Chapter 7
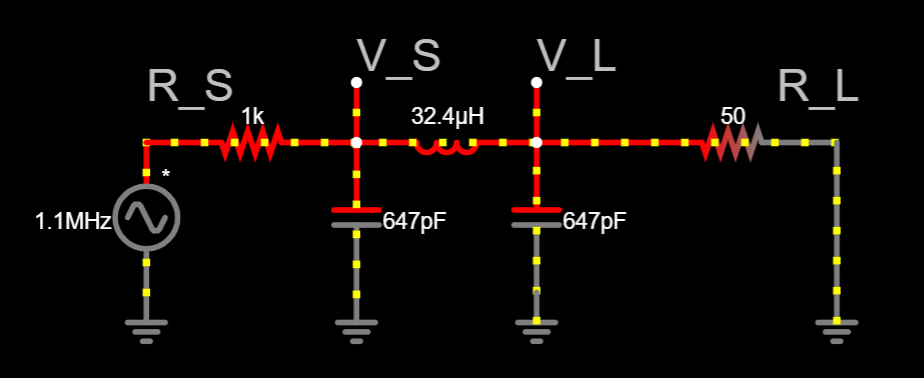
Maximum power transfer in AC
Condition: Z L ‾ = Z S ∗ ‾ Maximum power to load (50%): 2 P avg = P rms ⋅ 2 = P max = ∣ V S ∣ 2 4 R S = ∣ V L ∣ 2 4 R L Total maximum power: 2 P avg = P rms ⋅ 2 = P max = ∣ V S ∣ 2 2 R S
\begin{align}
\text{Condition: } &\overline{Z_L} = \overline{Z_S^*}\\
\text{Maximum power to load (50\%): } &2P_\text{avg}=P_\text{rms}\cdot\sqrt{2} = P_\text{max} = \frac{{|V_S|}^2}{4R_S}\\
& = \frac{{|V_L|}^2}{4R_L}\\
\text{Total maximum power: } &2P_\text{avg}=P_\text{rms}\cdot\sqrt{2} = P_\text{max} = \frac{{|V_S|}^2}{2R_S}
\end{align}
Condition: Maximum power to load (50%): Total maximum power: Z L = Z S ∗ 2 P avg = P rms ⋅ 2 = P max = 4 R S ∣ V S ∣ 2 = 4 R L ∣ V L ∣ 2 2 P avg = P rms ⋅ 2 = P max = 2 R S ∣ V S ∣ 2
Complex Power
Where V ˉ = V ∠ θ \bar{V}=V\angle\theta V ˉ = V ∠ θ I ˉ = I ∠ ϕ \bar{I}=I\angle\phi I ˉ = I ∠ ϕ
Complex [VA]: S ˉ = V ˉ rms × I ˉ rms ∗ = V ˉ × I ˉ ∗ 2 = V I 2 ∠ ( θ − ϕ ) Apparent [VA]: ∣ S ∣ Real [W]: P = ∣ S ∣ cos ( θ − ϕ ) = Re ( S ˉ ) Reactive [VAR]: Q = ∣ S ∣ cos ( θ − ϕ ) = Im ( S ˉ ) Q = P tan ( arccos ( Power factor ) ) Power Factor = P ∣ S ∣ = cos ( arctan ( Q P ) )
\begin{align}
\text{Complex [VA]: }\bar{S} &= \bar{V}_\text{rms}\times \bar{I}_\text{rms}^* = \frac{\bar{V}\times \bar{I}^*}{2} = \frac{VI}{2}\angle(\theta-\phi)\\
\text{Apparent [VA]: } |S|\\
\text{Real [W]: } P &= |S| \cos(\theta-\phi) = \text{Re}(\bar{S})\\
\text{Reactive [VAR]: } Q &= |S| \cos(\theta-\phi) = \text{Im}(\bar{S})\\
Q &= P\tan(\arccos(\text{Power factor}))\\
\text{Power Factor} &= \frac{P}{|S|} = \cos\left(\arctan\left(\frac{Q}{P}\right)\right)
\end{align}
Complex [VA]: S ˉ Apparent [VA]: ∣ S ∣ Real [W]: P Reactive [VAR]: Q Q Power Factor = V ˉ rms × I ˉ rms ∗ = 2 V ˉ × I ˉ ∗ = 2 V I ∠ ( θ − ϕ ) = ∣ S ∣ cos ( θ − ϕ ) = Re ( S ˉ ) = ∣ S ∣ cos ( θ − ϕ ) = Im ( S ˉ ) = P tan ( arccos ( Power factor )) = ∣ S ∣ P = cos ( arctan ( P Q ) )
Where S ˉ = ∣ S ∣ ∠ φ \bar{S}=|S|\angle\varphi S ˉ = ∣ S ∣∠ φ
φ = arctan ( Q P ) \varphi = \arctan\left(\frac{Q}{P}\right) φ = arctan ( P Q )
Lagging
Leading
Voltage
Current behind
Current ahead
Load type
Inductive
Capacitive
Q Q Q Q > 0 Q>0 Q > 0 Q < 0 Q<0 Q < 0
φ \varphi φ φ > 0 \varphi>0 φ > 0 φ < 0 \varphi<0 φ < 0
Chapter 8
Constants
μ 0 = 4 π × 1 0 − 7
\mu_0=4\pi \times 10^{-7}
μ 0 = 4 π × 1 0 − 7
Faraday’s law: ε = − N d Φ d t Ampere’s law: B = μ 0 I 2 π r
\begin{align}
\text{Faraday's law: }\varepsilon &= -N\frac{d\varPhi}{dt}\\
\text{Ampere's law: }B &= \frac{\mu_0 I}{2\pi r}\\
\end{align}
Faraday’s law: ε Ampere’s law: B = − N d t d Φ = 2 π r μ 0 I
Step up: n > 1 n>1 n > 1 n < 1 n<1 n < 1
V s V p = N s N p = i p i s = n Z ˉ i n = 1 n 2 Z ˉ L
\begin{align}
\frac{V_s}{V_p} &= \frac{N_s}{N_p}=\frac{i_p}{i_s}=n\\
\bar{Z}_{in} &= \frac{1}{n^2}\bar{Z}_L
\end{align}
V p V s Z ˉ in = N p N s = i s i p = n = n 2 1 Z ˉ L
Motor
For permanent motors, define permanent torque constant k T P = k T Φ k_{TP}=k_T\varPhi k TP = k T Φ
T = k T × Φ × i a = k T P × i a P mech = ω mech T = ω mech × k T P × i a
\begin{align}
T &= k_T\times\varPhi \times i_a = k_{TP} \times i_a\\
P_\text{mech} &= \omega_\text{mech} T = \omega_\text{mech} \times k_{TP}\times i_a
\end{align}
T P mech = k T × Φ × i a = k TP × i a = ω mech T = ω mech × k TP × i a
Back emf
Define for permanent motors, define permanent armature constant k a P = k a Φ k_{aP} = k_a\varPhi k a P = k a Φ v a v_a v a i a i_a i a
e b = k a × Φ × ω mech = k a P × ω mech
\begin{align}
e_b=k_a\times \varPhi\times \omega_\text{mech} = k_{aP} \times\omega_\text{mech}\\
\end{align}
e b = k a × Φ × ω mech = k a P × ω mech
Summary
For ideal motor, torque and armature constants are the same: k a = k T k_a=k_T k a = k T p p p M M M
Power dissipated: P e = e b × i a = k a P × ω mech × i a Constants for ideal motor: k a = k T = p N 2 π M
\begin{align}
\text{Power dissipated: } P_e &= e_b\times i_a = k_{aP} \times \omega_\text{mech} \times i_a\\
\text {Constants for ideal motor: } k_a &= k_T = \frac{pN}{2\pi M}
\end{align}
Power dissipated: P e Constants for ideal motor: k a = e b × i a = k a P × ω mech × i a = k T = 2 π M pN
For permanent magnet DC motor in DC steady state:b b b T L T_L T L
{ 0 = v a − i a R a − k a P ω mech = v a − i a R a − e b k T P i a = T L + b × ω mech Analog speed control (Voltage): T = k T P R v s − k T P k a P R ω mech Analog speed control (Current): T = k T P R S R i s − k T P k a P R ω mech
\begin{align}
&\begin{cases}
0 &= v_a - i_a R_a - k_{aP} \omega_\text{mech} = v_a - i_a R_a - e_b \\
k_{TP} i_a &= T_L + b\times \omega_\text{mech}
\end{cases}\\
&\text{Analog speed control (Voltage): } T = \frac{k_{TP}}{R}v_s - \frac{k_{TP}k_{aP}}{R} \omega_\text{mech}\\
&\text{Analog speed control (Current): } T = \frac{k_{TP}R_S}{R}i_s - \frac{k_{TP}k_{aP}}{R} \omega_\text{mech}
\end{align}
{ 0 k TP i a = v a − i a R a − k a P ω mech = v a − i a R a − e b = T L + b × ω mech Analog speed control (Voltage): T = R k TP v s − R k TP k a P ω mech Analog speed control (Current): T = R k TP R S i s − R k TP k a P ω mech